up
-
- source.nb
-
- source.cdf
-
- source code in HTML
-
- source code in PDF
Solving 2D Poisson PDE on non-uniform
rectangle grid
May 30, 2014 compiled on — Wednesday July 06, 2016 at 08:30 AM
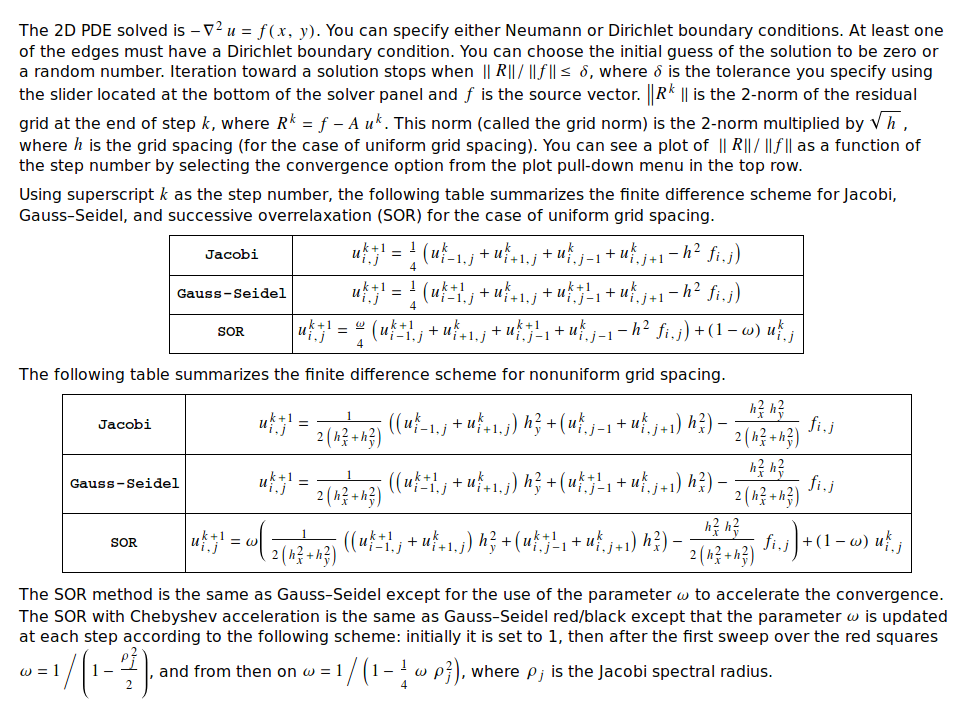
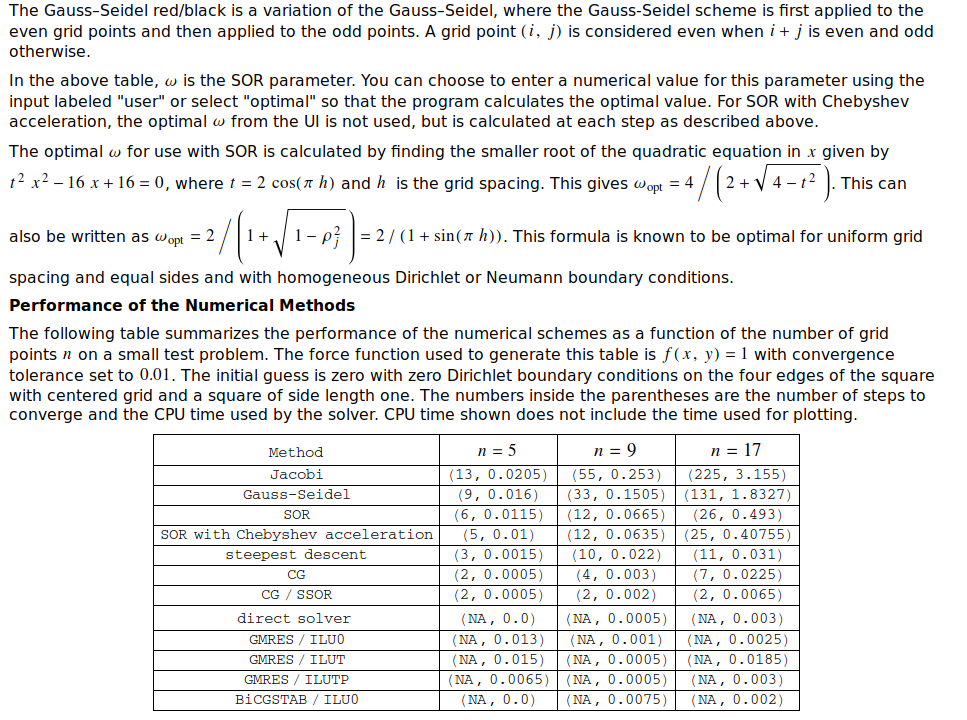
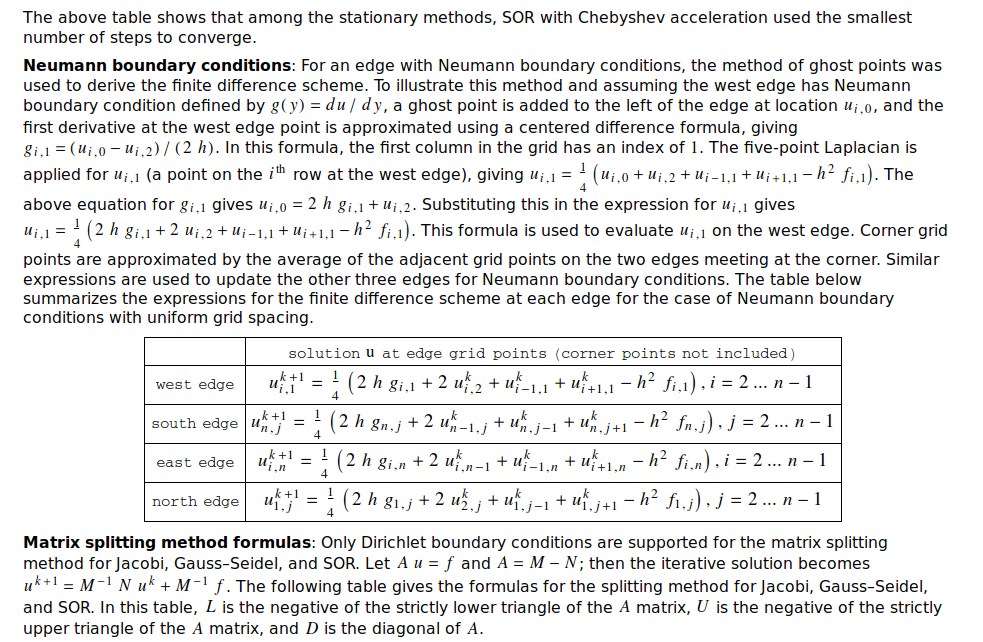
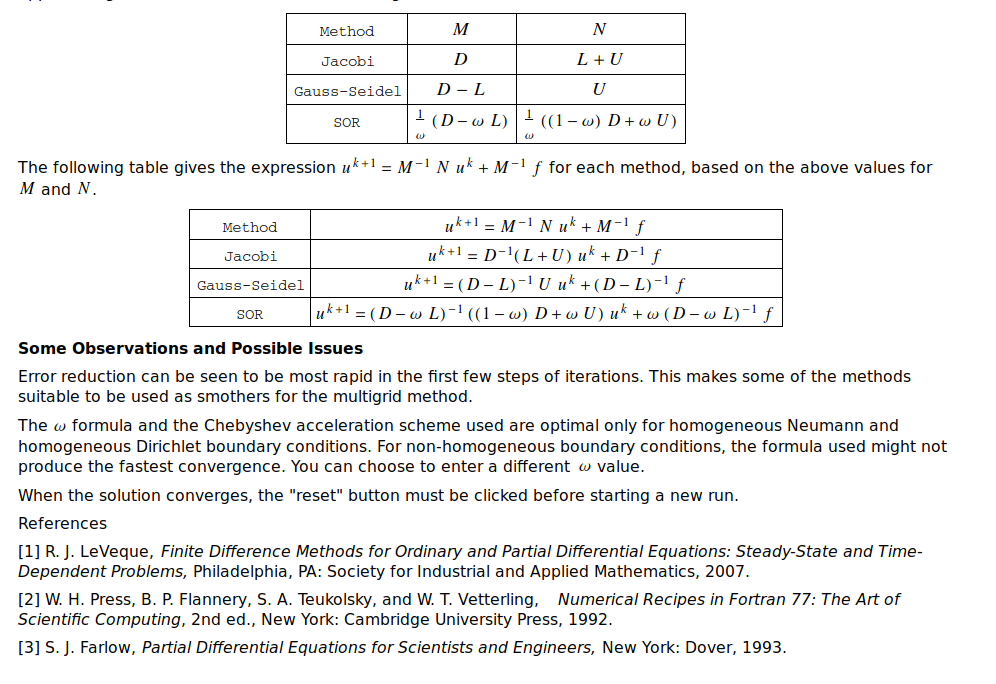
2D Poisson PDE on non-uniform rectangle grid.  . Boundary conditions supported are
Neummann and Dirichlet. Solved using the following methods:
. Boundary conditions supported are
Neummann and Dirichlet. Solved using the following methods:
direct, Jacobi, Gauss-Seidel, Gauss-Seidel red/black, SOR, Chebyshev, steepest descent, conjugate
gradient, GMRES, BiCGSTAB