#1

$y^{\prime }\left(x\right)-\frac{1}{\sqrt{a_4{x}^4+a_3{x}^3+a_2{x}^2+a_{1}x+a_0}}=0$

Maple

restart; 
ode1:=diff(y(x),x)-(a4*x^4+a3*x^3+a2*x^2+a1*x+a0)^(-1/2)=0; 
dsolve(%,y(x));

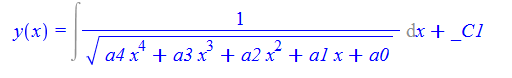
Mathematica

Remove["Global`*"] 
ode1=y'[x]-1/Sqrt[(a4 x^4+a3 x^3+a2 x^2+a1 x+a0)]==0; 
DSolve[ode1,y,x]

#2

$y^{\prime }\left(x\right)+ay\left(x\right)=c{e}^{bx}$
Maple

restart; 
ode2:=diff(y(x),x)+a*y(x)=c*exp(b*x); 
dsolve(%,y(x));

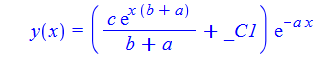
Mathematica

Remove["Global`*"] 
ode2=y'[x]+a y[x]-c Exp[b*x]==0 
DSolve[%,y[x],x]// TraditionalForm

#3

$y^{\prime }\left(x\right)+ay\left(x\right)-b\sin \left(cx\right)=0$
Maple

restart; 
ode3:=diff(y(x),x)+a*y(x)-b*sin(c*x)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode3=y'[x]+a y[x]-b Sin[c x]==0; 
DSolve[%,y[x],x]// TraditionalForm

#4

$y^{\prime }\left(x\right)+2xy\left(x\right)-x{e}^{-x^2}=0$

Maple

restart; 
ode4:=diff(y(x),x)+2*x*y(x)-x*exp(-x^2)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode4=y'[x]+2 x y[x]-x Exp[-x^2]==0; 
DSolve[%,y[x],x]// TraditionalForm

#5

$y^{\prime }\left(x\right)+y\left(x\right)\cos \left(x\right)-e^{2x}=0$

Maple

restart; 
ode5:=diff(y(x),x)+y(x)*cos(x)-exp(2*x)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode5=y'[x]+y[x] Cos[x]-Exp[2*x]==0; 
DSolve[%,y[x],x]// TraditionalForm

#6

$y^{\prime }\left(x\right)+y\left(x\right)\cos \left(x\right)-\frac{1}{2}\sin \left(2x\right)=0$

Maple

restart; 
ode6:=diff(y(x),x)+y(x)*cos(x)-1/2*sin(2*x)=0: 
dsolve(%,y(x));

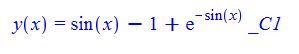
Mathematica

Remove["Global`*"] 
ode6=y'[x]+y[x] Cos[x]-1/2 Sin[2 x]==0; 
DSolve[%,y[x],x]// TraditionalForm

#7

$y^{\prime }\left(x\right)+y\left(x\right)\cos \left(x\right)-e^{-\sin \left(x\right)}=0$

Maple

restart; 
ode7:=diff(y(x),x)+y(x)*cos(x)-exp(-sin(x))=0: 
dsolve(%,y(x));

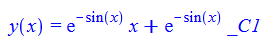
Mathematica

Remove["Global`*"] 
ode7=y'[x]+y[x] Cos[x]-Exp[-Sin[x]]==0; 
DSolve[%,y[x],x]// TraditionalForm

#8

$y^{\prime }\left(x\right)+y\left(x\right)\tan \left(x\right)-\sin \left(2x\right)=0$

Maple

restart; 
ode8:=diff(y(x),x)+y(x)*tan(x)-sin(2*x)=0: 
dsolve(%,y(x));

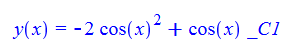
Mathematica

Remove["Global`*"] 
ode8=y'[x]+y[x] Tan[x]-Sin[2 x]==0; 
DSolve[%,y[x],x]// TraditionalForm

#9

$y^{\prime }\left(x\right)-(\sin (\ln \left(x\right))+\cos (\ln \left(x\right))+a)y\left(x\right)=0$

Maple

restart; 
ode9:=diff(y(x),x)-(sin(log(x))+cos(log(x))+a)*y(x)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode9=y'[x]-(Sin[Log[x]]+Cos[Log[x]]+a) y[x]==0; 
DSolve[%,y[x],x]// TraditionalForm

#10

$y^{\prime }\left(x\right)+f^{\prime }\left(x\right)y\left(x\right)-f\left(x\right)f^{\prime }\left(x\right)=0$

Maple

restart; 
ode10:=diff(y(x),x)+diff(f(x),x)*y(x)=f(x)*diff(f(x),x); 
dsolve(%,y(x));

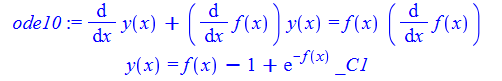
Mathematica

Remove["Global`*"] 
ode10=y'[x]+f'[x]*y[x]-f[x]*f'[x]==0; 
DSolve[%,y[x],x]// TraditionalForm

#11

$y^{\prime }\left(x\right)+f\left(x\right)y\left(x\right)=g\left(x\right)$

Maple

restart; 
ode11:=diff(y(x),x)+f(x)*y(x)=g(x); 
dsolve(%,y(x));

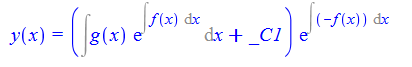
Mathematica

Remove["Global`*"] 
ode11=y'[x]+f[x] y[x]==g[x]; 
First[DSolve[%,y[x],x]]// TraditionalForm

#12

$y^{\prime }\left(x\right)+y{\left(x\right)}^2=1$

Maple

restart; 
ode12:=diff(y(x),x)+(y(x))^2=1: 
dsolve(%,y(x));

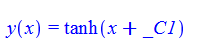
Mathematica

Remove["Global`*"] 
ode12=y'[x]+y[x]^2==1; 
DSolve[%,y[x],x]// TraditionalForm 
ExpToTrig[%]//FullSimplify// TraditionalForm

#13

$y^{\prime }\left(x\right)+y{\left(x\right)}^2=ax+b$

Maple

restart; 
ode13:=diff(y(x),x)+(y(x))^2=a*x+b: 
dsolve(%,y(x));

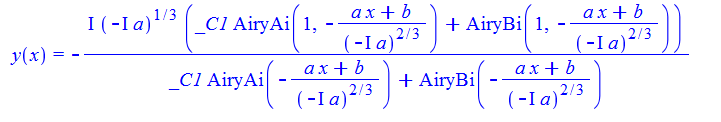
Mathematica

Remove["Global`*"] 
ode13=y'[x]+y[x]^2==a x+b; 
DSolve[%,y[x],x]// TraditionalForm

#14

$y^{\prime }\left(x\right)+y{\left(x\right)}^2+a{x}^m=0$

Maple

restart; 
ode14:=diff(y(x),x)+(y(x))^2+a*x^m=0: 
dsolve(%,y(x));

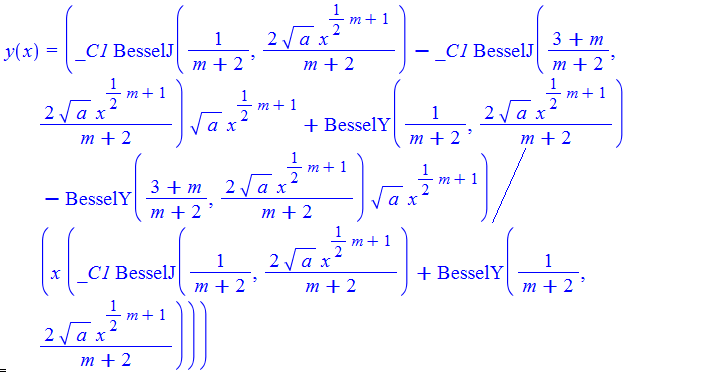
Mathematica

Remove["Global`*"] 
ode14=y'[x]+y[x]^2+a x^m==0; 
DSolve[%,y[x],x]// TraditionalForm

#15

$y^{\prime }\left(x\right)+y{\left(x\right)}^2-2x^{2}y\left(x\right)+x^4-2x-1=0$

Maple

restart; 
ode15:=diff(y(x),x)+(y(x))^2-2*x^2*y(x)+x^4-2*x-1=0: 
dsolve(%,y(x));

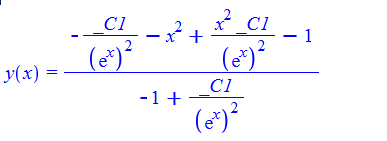
Mathematica

Remove["Global`*"] 
ode15 = y'[x] + y[x]^2 - 2 x^2 y[x] + x^4 - 2 x - 1 == 0; 
DSolve[%, y[x], x]// TraditionalForm

#16

$y^{\prime }\left(x\right)+y{\left(x\right)}^2-(xy\left(x\right)-1)f\left(x\right)=0$

Maple

restart; 
ode16:=diff(y(x),x)+y(x)^2- (x*y(x)-1)*f(x)=0: 
dsolve(%,y(x));

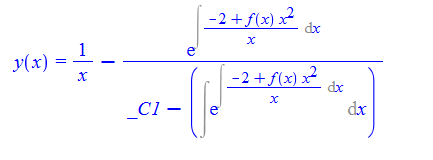
Mathematica

Remove["Global`*"] 
ode16 = y'[x] + y[x]^2 + (x*y[x] - 1)*f[x] == 0; 
DSolve[%, y[x], x]

#17

$y^{\prime }\left(x\right)-y{\left(x\right)}^2-3y\left(x\right)+4=0$

Maple

restart; 
ode16:=diff(y(x),x)-y(x)^2- 3*y(x)+4=0: 
dsolve(%,y(x));

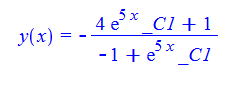
Mathematica

Remove["Global`*"] 
ode17 = y'[x] - y[x]^2 - 3*y[x] + 4 == 0; 
DSolve[%, y[x], x]// TraditionalForm

#18

$y^{\prime }\left(x\right)-y{\left(x\right)}^2-xy\left(x\right)-x+1=0$

Maple

restart; 
ode18:=diff(y(x),x)-y(x)^2- x*y(x)-x+1=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode18 = y'[x] - y[x]^2 - x*y[x] - x + 1 == 0; 
DSolve[%, y[x], x]// TraditionalForm

#19

$y^{\prime }\left(x\right)-{(y\left(x\right)+x)}^2=0$

Maple

restart; 
ode19:=diff(y(x),x)-(y(x)+x)^2=0: 
dsolve(%,y(x));

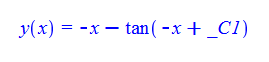
Mathematica

Remove["Global`*"] 
ode19=y'[x]-(y[x]+x)^2==0; 
DSolve[%,y[x],x]// TraditionalForm

#20

$y^{\prime }\left(x\right)-y{\left(x\right)}^2+(x^2+1)y\left(x\right)-2x=0$

Maple

restart; 
ode20:=diff(y(x),x)-y(x)^2+(x^2+1)*y(x)-2*x=0: 
dsolve(%,y(x));

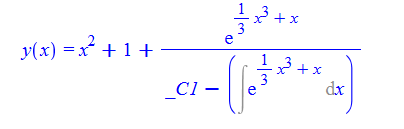
Mathematica

Remove["Global`*"] 
ode20=y'[x]-y[x]^2+(x^2+1)*y[x]-2*x==0; 
DSolve[%,y[x],x]// TraditionalForm

#21

$y^{\prime }\left(x\right)-y{\left(x\right)}^2+y\left(x\right)\sin \left(x\right)-\cos \left(x\right)=0$

Maple

restart; 
ode21:=diff(y(x),x)-y(x)^2+y(x)*sin(x)-cos(x)=0: 
dsolve(%,y(x));

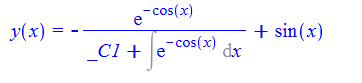
Mathematica

Remove["Global`*"] 
ode21=y'[x]-y[x]^2+y[x]*Sin[x]-Cos[x]==0; 
DSolve[%,y[x],x]// TraditionalForm

#22

$y^{\prime }\left(x\right)-y{\left(x\right)}^2-y\left(x\right)\sin \left(2x\right)-\cos \left(2x\right)=0$

Maple

restart; 
ode22:=diff(y(x),x)-sin(2*x)*y(x)-y(x)^2=0; 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode22=y'[x]-y[x]^2-y[x]*Sin[2*x]-Cos[2*x]==0 
DSolve[%,y[x],x]

#23

$y^{\prime }\left(x\right)+ay{\left(x\right)}^2-b=0$

Maple

restart; 
ode23:=diff(y(x),x)+a*y(x)^2-b=0: 
dsolve(%,y(x));

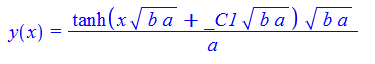
Mathematica

Remove["Global`*"] 
ode23=y'[x]+a*y[x]^2-b==0; 
DSolve[%,y[x],x]// TraditionalForm

#24

$y^{\prime }\left(x\right)+ay{\left(x\right)}^2-b{x}^{\nu }=0$

Maple

restart; 
ode24:=diff(y(x),x)+a*y(x)^2-b*x^nu=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode24=y'[x]+a*y[x]^2-b*x^nu==0; 
DSolve[%,y[x],x]//TraditionalForm

#25

$y^{\prime }\left(x\right)+ay{\left(x\right)}^2-b{x}^{2\nu }-c{x}^{\nu -1}=0$

Maple

restart; 
ode25:=diff(y(x),x)+a*y(x)^2-b*x^(2*nu)-c*x^(nu-1)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode25=y'[x]+a*y[x]^2-b*x^(2*\[Nu])-c*x^(\[Nu]-1)==0 
DSolve[%,y[x],x]// Simplify // TraditionalForm

#26

$y^{\prime }\left(x\right)-(Ay\left(x\right)-a)(By\left(x\right)-b)=0$

Maple

restart; 
ode26:=diff(y(x),x)-(A*y(x)-a)*(B*y(x)-b)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode26=y'[x]-(A*y[x]-a)*(B*y[x]-b)==0; 
DSolve[%,y[x],x]//TraditionalForm

#27

$y^{\prime }\left(x\right)+ay\left(x\right)(y\left(x\right)-x)-1=0$

Maple

restart; 
ode27:=diff(y(x),x)+a*y(x)*(y(x)-x)-1=0: 
dsolve(%,y(x));

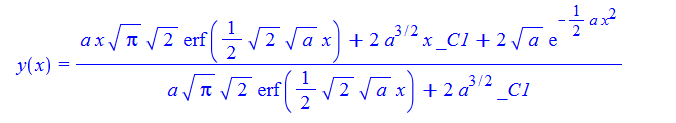
Mathematica

Remove["Global`*"] 
ode27=y'[x]+a*y[x]*(y[x]-x)-1==0; 
DSolve[%,y[x],x]//TraditionalForm

#28

$y^{\prime }\left(x\right)+xy{\left(x\right)}^2-x^{3}y\left(x\right)-2x=0$

Maple

restart; 
ode28:=diff(y(x),x)+x*y(x)^2-x^3*y(x)-2*x=0; 
dsolve(%,y(x));

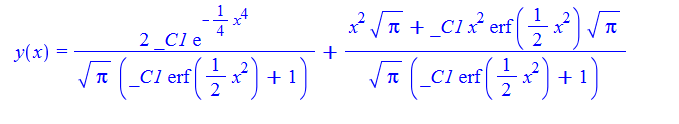
Mathematica

Remove["Global`*"] 
ode28=y'[x]+x*y[x]^2-x^3*y[x]-2*x==0; 
DSolve[%,y[x],x]//TraditionalForm

#29

$y^{\prime }\left(x\right)-xy{\left(x\right)}^2-3xy\left(x\right)=0$

Maple

restart; 
ode29:=diff(y(x),x)-x*y(x)^2-3*x*y(x)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode29=y'[x]-x*y[x]^2-3*x*y[x]==0; 
DSolve[%,y[x],x]//TraditionalForm

#30

$y^{\prime }\left(x\right)+x^{(-1-a)}y{\left(x\right)}^2-x^a=0$

Maple

restart; 
ode30:=diff(y(x),x)+x^(-1-a)*y(x)^2-x^a=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode30=y'[x]+x^(-a-1)*y[x]^2-x^a==0 
DSolve[%,y[x],x]

#31

$y^{\prime }\left(x\right)-a{x}^n(y{\left(x\right)}^2+1)=0$

Maple

restart; 
ode31:=diff(y(x),x)-a*x^n*(y(x)^2+1)=0: 
dsolve(%,y(x));

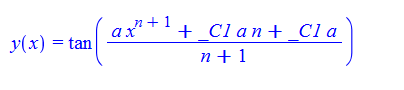
Mathematica

Remove["Global`*"] 
ode31 = y'[x] - a*x^n*(y[x]^2 + 1) == 0; 
DSolve[%, y[x], x] // TraditionalForm

#32

$y^{\prime }\left(x\right)+y{\left(x\right)}^2\sin \left(x\right)-2\frac{\sin \left(x\right)}{\cos {\left(x\right)}^2}=0$

Maple

restart; 
ode31:=diff(y(x),x)+y(x)^2*sin(x)-2*sin(x)/cos(x)^2=0: 
dsolve(%,y(x));

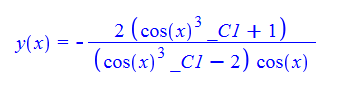
Mathematica

Remove["Global`*"] 
ode32=y'[x]+y[x]^2*Sin[x]-2*Sin[x]/Cos[x]^2==0; 
DSolve[%,y[x],x]//TraditionalForm

#33

$y^{\prime }\left(x\right)-\frac{y(x{)}^2{f}^{\prime }\left(x\right)}{g\left(x\right)}+\frac{g^{\prime }\left(x\right)}{f\left(x\right)}=0$

Maple

restart; 
ode33:=diff(y(x),x)-y(x)^2*diff(f(x),x)/g(x)+diff(g(x),x)/f(x)=0; 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
(ode33=y'[x]-y[x]^2*D[f[x],x]/g[x]+D[g[x],x]/f[x]==0)//TraditionalForm 
DSolve[ode33,y[x],x]//TraditionalForm

#34

$y^{\prime }\left(x\right)+f\left(x\right)y{\left(x\right)}^2+g\left(x\right)y\left(x\right)=0$

Maple

restart; 
ode34:=diff(y(x),x)+f(x)*y(x)^2+g(x)*y(x)=0: 
dsolve(%,y(x));

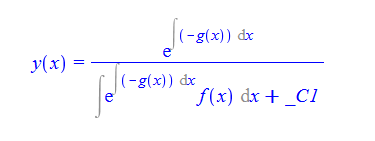
Mathematica

Remove["Global`*"] 
ode34=y'[x]+f[x]*y[x]^2+g[x]*y[x]==0 
DSolve[%,y[x],x]//TraditionalForm

#35

$y^{\prime }\left(x\right)+f\left(x\right)(y{\left(x\right)}^2+2ay\left(x\right)+b)=0$

Maple

restart; 
ode35:=diff(y(x),x)+f(x)*(y(x)^2+2*a*y(x)+b)=0: 
dsolve(%,y(x));

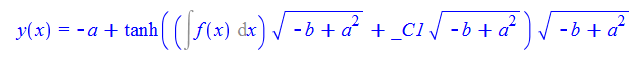
Mathematica

Remove["Global`*"] 
ode35 = y'[x] + f[x]*(y[x]^2 + 2*a*y[x] + b) == 0; 
DSolve[%, y[x], x] // TraditionalForm

#36

$y^{\prime }\left(x\right)+y{\left(x\right)}^3+axy{\left(x\right)}^2=0$

Maple

restart; 
ode36:=diff(y(x),x)+y(x)^3+a*x*y(x)^2=0: 
dsolve(%,y(x));

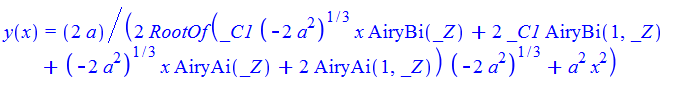
Mathematica

Remove["Global`*"] 
ode36 = y'[x] + y[x]^3 + a*x*y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#37

$y^{\prime }\left(x\right)-y{\left(x\right)}^3-a{e}^{x}y{\left(x\right)}^2=0$

Maple

restart; 
ode37:=diff(y(x),x)-y(x)^3-a*exp(x)*y(x)^2=0: 
dsolve(%,y(x));

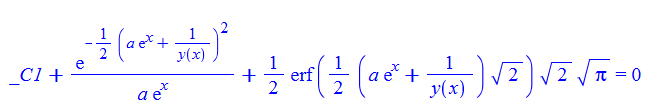
Mathematica

Remove["Global`*"] 
ode37 = y'[x] - y[x]^3 - a*Exp[x]*y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#38

$y^{\prime }\left(x\right)-ay{\left(x\right)}^3-b{x}^{\frac{3}{2}}=0$

Maple

restart; 
ode38:=diff(y(x),x)-a*y(x)^3-b*x^(3/2)=0: 
dsolve(%,y(x));

Nothing returned

Mathematica

Remove["Global`*"] 
ode38 = y'[x] - a*y[x]^3 - b*x^(3/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#39

$y^{\prime }\left(x\right)-a_{3}y{\left(x\right)}^3-a_{2}y{\left(x\right)}^2-a_{1}y\left(x\right)-a_0=0$

Maple

restart; 
ode39:=diff(y(x),x)-a3*y(x)^3-a2*y(x)^2-a1*y(x)-a0=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode39 = y'[x] - a3*y[x]^3 - a2*y[x]^2 - a1*y[x] - a0 == 0 
DSolve[%, y[x], x] // TraditionalForm

#40

$y^{\prime }\left(x\right)+3ay{\left(x\right)}^3+6axy{\left(x\right)}^2=0$

Maple

restart; 
ode40:=diff(y(x),x)+3*a*y(x)^3+6*a*x*y(x)^2=0: 
dsolve(%,y(x));

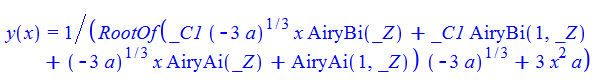
Mathematica

Remove["Global`*"] 
ode40 = y'[x] + 3*a*y[x]^3 + 6*a*x*y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#41

$y^{\prime }\left(x\right)+axy{\left(x\right)}^3+by{\left(x\right)}^2=0$

Maple

restart; 
ode41:=diff(y(x),x)+a*x*y(x)^3+b*y(x)^2=0: 
dsolve(%,y(x));

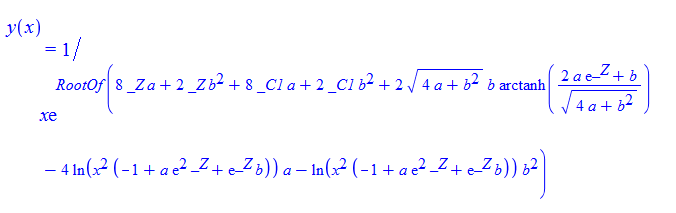
Mathematica

Remove["Global`*"] 
ode41 = y'[x] + a*x*y[x]^3 + b*y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#42

$y^{\prime }\left(x\right)-x(x+2)y{\left(x\right)}^3-(x+3)y{\left(x\right)}^2=0$

Maple

restart; 
ode42:=diff(y(x),x)-x*(x+2)*y(x)^3-(x+3)*y(x)^2=0: 
dsolve(%,y(x));

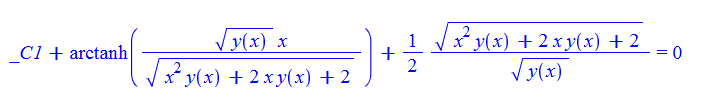
Mathematica

Remove["Global`*"] 
ode42 = y'[x] - x*(x + 2)*y[x]^3 - (x + 3)*y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#43

$y^{\prime }\left(x\right)+(3a{x}^2+4a^{2}x+b)y{\left(x\right)}^3+3xy{\left(x\right)}^2=0$

Maple

restart; 
ode43:=diff(y(x),x)+(3*a*x^2+4*a^2*x+b)*y(x)^3+3*x*y(x)^2=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode43 = y'[x] + (3*a*x^2 + 4*a^2*x + b)*y[x]^3 + 3*x*y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#44

$y^{\prime }\left(x\right)+2a{x}^{3}y{\left(x\right)}^3+2xy\left(x\right)=0$

Maple

restart; 
ode44:=diff(y(x),x)+2*a*x^3*y(x)^3+2*x*y(x)=0: 
dsolve(%,y(x));

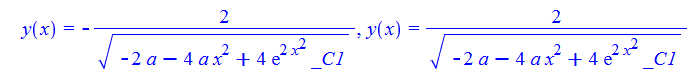
Mathematica

Remove["Global`*"] 
ode44 = y'[x] + 2*a*x^3*y[x]^3 + 2*x*y[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#45

$y^{\prime }\left(x\right)+2(a^2{x}^3-b^{2}x)y{\left(x\right)}^3+3by{\left(x\right)}^2=0$

Maple

restart; 
ode45:=diff(y(x),x)+2*(a^2*x^3-b^2*x)*y(x)^3+3*b*y(x)^2=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode45 = y'[x] + 2*(a^2*x^3 - b^2*x)*y[x]^3 + 3*b*y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#46

$y^{\prime }\left(x\right)-x^{a}y{\left(x\right)}^3+3y{\left(x\right)}^2-x^{-a}y\left(x\right)-x^{-2a}+a{x}^{-a-1}=0$

Maple

restart; 
ode46:=diff(y(x),x)-x^a*y(x)^3+3*y(x)^2-x^(-a)*y(x)-x^(-2*a)+a*x^(-a-1)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode46=y'[x]-x^a*y[x]^3+3*y[x]^2-x^(-a)*y[x]-x^(-2*a)+a*x^(-a-1)==0 
DSolve[%, y[x], x] // TraditionalForm

#47

$y^{\prime }\left(x\right)-a(x^n-x)y{\left(x\right)}^3-y{\left(x\right)}^2=0$

Maple

restart; 
ode47:=diff(y(x),x)-a*(x^n-x)*y(x)^3-y(x)^2=0: 
dsolve(%,y(x));

No output

Mathematica

Remove["Global`*"] 
ode47 = y'[x] - a*(x^n - x)*y[x]^3 - y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#48

$y^{\prime }\left(x\right)-(a{x}^n+bx)y{\left(x\right)}^3-cy{\left(x\right)}^2=0$

Maple

restart; 
ode48:=diff(y(x),x)-(a*x^n+b*x)*y(x)^3-c*y(x)^2=0: 
dsolve(%,y(x));

No output
Mathematica

Remove["Global`*"] 
ode48 = y'[x] - (a*x^n + b*x)*y[x]^3 - c*y[x]^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#49

$y^{\prime }\left(x\right)+a{\varphi }^{\prime }\left(x\right)y{\left(x\right)}^3+6a\varphi \left(x\right)y{\left(x\right)}^2+(2a+1)y\left(x\right)\frac{{\varphi }^{\prime \prime }\left(x\right)}{{\varphi }^{\prime }\left(x\right)}+2a+2=0$

Maple

restart; 
ode49:=diff(y(x),x)+a*diff(phi(x),x)*y(x)^3+6*a*phi(x)*y(x)^2+(2*a+1)*y(x)*diff(phi(x),x$2)/diff(phi(x),x)+2*a+2=0; 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode49 = y'[x] + a*phi'[x]*y[x]^3 + 
   6*a*phi[x]*y[x]^2 + (2*a + 1)*y[x]*phi''[x]/phi'[x] + 2*a + 2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#50

$y^{\prime }\left(x\right)-f_3\left(x\right)y{\left(x\right)}^3-f_2\left(x\right)y{\left(x\right)}^2-f_1\left(x\right)y\left(x\right)-f_0\left(x\right)=0$
Maple

restart; 
ode50:=diff(y(x),x)-f3(x)*y(x)^3-f2(x)*y(x)^2-f1(x)*y(x)-f0(x)=0; 
dsolve(%,y(x));

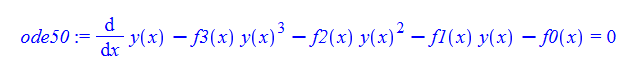
Mathematica

Remove["Global`*"] 
ode50 = y'[x] - f3[x]*y[x]^3 - f2[x]*y[x]^2 - f1[x]*y[x] - f0[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#51

$y^{\prime }\left(x\right)-(y\left(x\right)-f\left(x\right))(y\left(x\right)-g\left(x\right))(y\left(x\right)-\frac{af\left(x\right)+bg\left(x\right)}{a+b})h\left(x\right)-\frac{f^{\prime }\left(x\right)(y\left(x\right)-g\left(x\right))}{f\left(x\right)-g\left(x\right)}-\frac{g^{\prime }\left(x\right)(y\left(x\right)-f\left(x\right))}{g\left(x\right)-f\left(x\right)}=0$
Maple

restart; 
ode51:=diff(y(x),x)-(y(x)-f(x))*(y(x)-g(x))*(y(x)-(a*f(x)+b*g(x))/(a+b))*h(x)- 
diff(f(x),x)*(y(x)-g(x))/(f(x)-g(x))-diff(g(x),x)*(y(x)-f(x))/(g(x)-f(x))=0; 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode51 = D[y[x],x] - (y[x] - f[x])*(y[x] -g[x])*(y[x] - (a*f[x] + b*g[x])/(a + b))*h[x] - 
   D[f[x], x]*(y[x] - g[x])/(f[x] - g[x])-D[g[x], x]*(y[x] - f[x])/(g[x] - f[x]) == 0 
DSolve[%, y[x], x] // TraditionalForm

#52

$y^{\prime }\left(x\right)-b{x}^{\frac{n}{1-n}}-ay{\left(x\right)}^n=0$
Maple

restart; 
ode52:=diff(y(x),x)-b*x^(n/(1-n))-a*y(x)^n=0; 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode52 = D[y[x], x] - a*y[x]^n - b*x^(n/(1 - n)) == 0 
DSolve[%, y[x], x] // TraditionalForm

#53

$y^{\prime }\left(x\right)-\frac{y\left(x\right)f^{\prime }\left(x\right)}{f\left(x\right)}-f\left(x\right)g^{\prime }\left(x\right)-f{\left(x\right)}^{1-n}{(b+ag\left(x\right))}^{-n}y{\left(x\right)}^n{g}^{\prime }\left(x\right)=0$
Maple

restart; 
ode53:=diff(y(x),x)-(y(x)*diff(f(x),x))/f(x)-f(x)*diff(g(x),x)- 
f(x)^(1-n)/(b+a*g(x))^n*y(x)^n*diff(g(x),x)=0; 
dsolve(%,y(x));

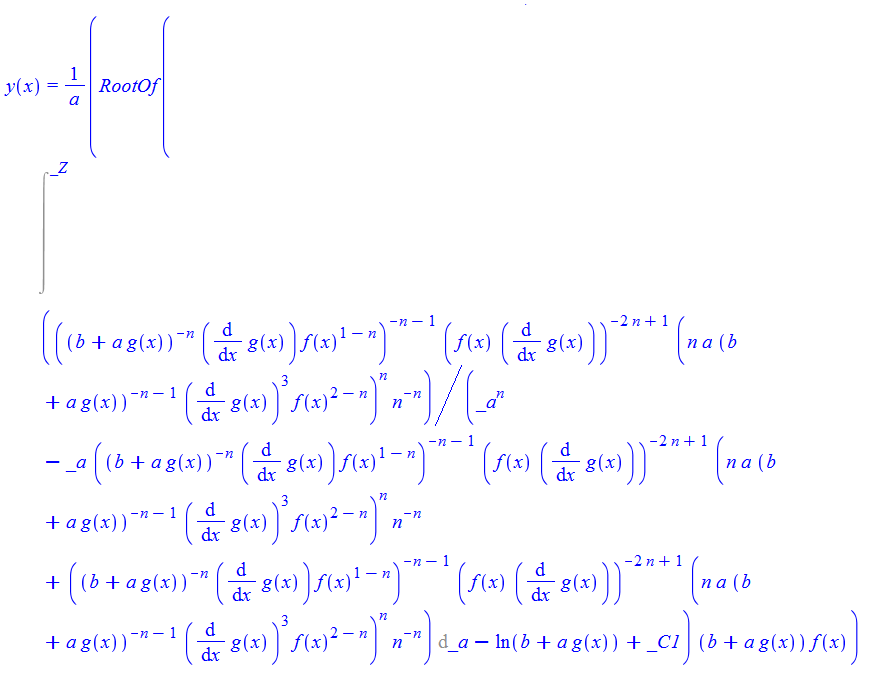
Mathematica

Remove["Global`*"] 
ode53 = y'[x] - f[x]^(1 - n)*g'[x]*y[x]^n/(a*g[x] + b)^n - 
   f'[x]*y[x]/f[x] - f[x]*g'[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#54

$y^{\prime }\left(x\right)-a^{n}f{\left(x\right)}^{1-n}y{\left(x\right)}^n{g}^{\prime }\left(x\right)-\frac{y\left(x\right)f^{\prime }\left(x\right)}{f\left(x\right)}-f\left(x\right)g^{\prime }\left(x\right)=0$
Maple

restart; 
ode54:=diff(y(x),x)-a^n*f(x)^(1-n)*diff(g(x),x)*y(x)^n-(diff(f(x),x)*y(x))/f(x)-f(x)*diff(g(x),x)=0: 
dsolve(%,y(x));

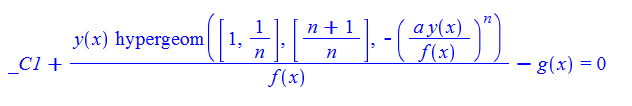
Mathematica

Remove["Global`*"] 
ode54=y'[x]-a^n*f[x]^(1-n)*g'[x]*y[x]^n-f'[x]*y[x]/f[x]-f[x]*g'[x]==0 
DSolve[%,y[x],x]//TraditionalForm

#55

$y^{\prime }\left(x\right)-f\left(x\right)y{\left(x\right)}^n-g\left(x\right)y\left(x\right)-h\left(x\right)=0$
Maple

restart; 
ode55:=diff(y(x),x)-f(x)*y(x)^n-g(x)*y(x)-h(x)=0: 
dsolve(%,y(x));

No answer

Mathematica

Remove["Global`*"] 
ode55 = y'[x] - f[x]*y[x]^n - g[x]*y[x] - h[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#56

$y^{\prime }\left(x\right)-f\left(x\right)y{\left(x\right)}^a-g\left(x\right)y{\left(x\right)}^b=0$
Maple

restart; 
ode56:=diff(y(x),x)-f(x)*y(x)^a-g(x)*y(x)^b=0: 
dsolve(%,y(x));

No answer

Mathematica

Remove["Global`*"] 
ode56=y'[x]-f[x]*y[x]^a-g[x]*y[x]^b==0 
DSolve[%,y[x],x]//TraditionalForm

#57

$y^{\prime }\left(x\right)-\sqrt{\left|y\left(x\right)\right|}=0$
Maple

restart; 
ode57:=diff(y(x),x)-sqrt(abs(y(x)))=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode57=y'[x]-Abs[y[x]]^(1/2)==0 
DSolve[%,y[x],x]//TraditionalForm

#58

$y^{\prime }\left(x\right)-ay{\left(x\right)}^{\frac{1}{2}}-bx=0$
Maple

restart; 
ode58:=diff(y(x),x)-a*y(x)^(1/2)-b*x=0: 
dsolve(%,y(x));

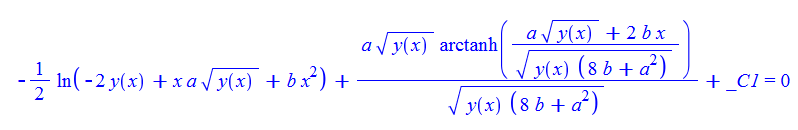
Mathematica

Remove["Global`*"] 
ode58 = y'[x] - a*y[x]^(1/2) - b*x == 0 
DSolve[%, y[x], x] // TraditionalForm

#59

$y^{\prime }\left(x\right)-a{(y{\left(x\right)}^2+1)}^{\frac{1}{2}}-b=0$
Maple

restart; 
ode59:=diff(y(x),x)-a*(y(x)^2+1)^(1/2)-b=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode59 = y'[x] - a*(y[x]^2 + 1)^(1/2) - b == 0 
DSolve[%, y[x], x] // TraditionalForm

#60

$y^{\prime }\left(x\right)-\frac{{(y{\left(x\right)}^2-1)}^{\frac{1}{2}}}{{(x^2-1)}^{\frac{1}{2}}}=0$
Maple

restart; 
ode60:=diff(y(x),x)-(y(x)^2-1)^(1/2)/(x^2-1)^(1/2)=0: 
dsolve(%,y(x)); 

Mathematica

Remove["Global`*"] 
ode60 = y'[x] - (y[x]^2 - 1)^(1/2)/(x^2 - 1)^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#61

$y^{\prime }\left(x\right)-\frac{{(x^2-1)}^{\frac{1}{2}}}{{(y{\left(x\right)}^2-1)}^{\frac{1}{2}}}=0$
Maple

restart; 
ode61:=diff(y(x),x)-(x^2-1)^(1/2)/(y(x)^2-1)^(1/2)=0: 
dsolve(%,y(x));

Mathematica

Remove["Global`*"] 
ode61 = y'[x] - (x^2 - 1)^(1/2)/(y[x]^2 - 1)^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#62

$y^{\prime }\left(x\right)-\frac{y\left(x\right)-x^2\sqrt{x^2-y{\left(x\right)}^2}}{x+xy\left(x\right)\sqrt{x^2-y{\left(x\right)}^2}}=0$
Maple

restart; 
ode62:=diff(y(x),x)-(y(x)-x^2*(x^2-y(x)^2)^(1/2))/(x+x*y(x)*(x^2-y(x)^2)^(1/2))=0: 
dsolve(%,y(x));

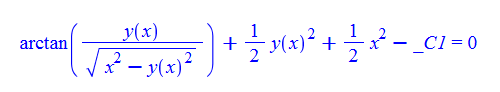
Mathematica

Remove["Global`*"] 
ode62 = D[y[x],x] - (y[x] - 
       x^2*(x^2 - y[x]^2)^(1/2))/(x*y[x]*(x^2 - y[x]^2)^(1/2) + x)==0; 
DSolve[%, y[x], x] // TraditionalForm

#63

$y^{\prime }\left(x\right)-\frac{1+y{\left(x\right)}^2}{{(1+x)}^{3/2}|y\left(x\right)+\sqrt{1+y\left(x\right)}|}=0$
Maple

restart; 
ode63:=diff(y(x),x)-(1+y(x)^2)/((1+x)^(3/2)*abs(y(x)+sqrt(1+y(x))))=0: 
dsolve(%,y(x));

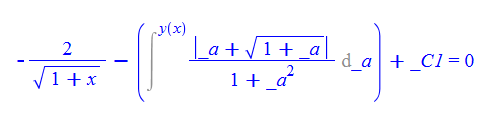
Mathematica

Remove["Global`*"]; 
ode63 = D[y[x],x]-(y[x]^2 + 1)/(Abs[y[x]+(1+y[x])^(1/2)]*(1+x)^(3/2))==0 
DSolve[%, y[x], x] // TraditionalForm

No answer after 10 minutes wait

#64

$y^{\prime }\left(x\right)-\sqrt{\frac{c+by\left(x\right)+ay{\left(x\right)}^2}{c+bx+a{x}^2}}=0$
Maple

restart; 
ode64:=diff(y(x),x)-sqrt((c+b*y(x)+a*y(x)^2)/(c+b*x+a*x^2))=0: 
dsolve(%,y(x));

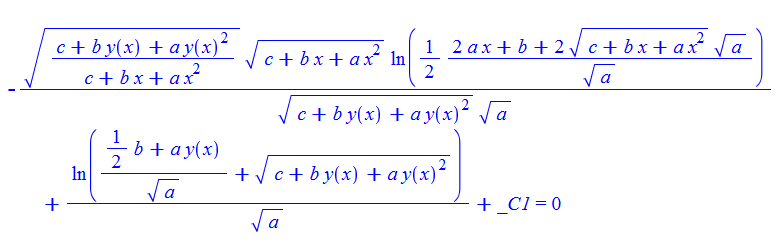
Mathematica

Clear["Global`*"]; 
ode64 =D[y[x], x] - ((a*y[x]^2 + b*y[x] + c)/(a*x^2 + b*x + c))^(1/2)==0 
DSolve[%, y[x], x] // TraditionalForm

#65

$y^{\prime }\left(x\right)-\sqrt{\frac{1+y{\left(x\right)}^3}{1+x^3}}=0$
Maple

restart; 
ode65:=diff(y(x),x)-sqrt((1+y(x)^3)/(1+x^3))=0: 
dsolve(%,y(x));

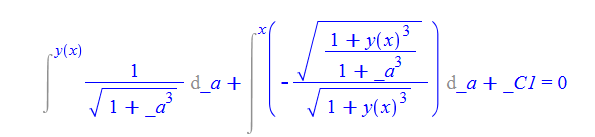
Mathematica

Clear["Global`*"]; 
ode65 = D[y[x], x] - ((y[x]^3 + 1)/(x^3 + 1))^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#66

$y^{\prime }\left(x\right)-\sqrt{\frac{|(1-y\left(x\right))y\left(x\right)(1-ay\left(x\right))|}{\left|(1-x)x(1-ax)\right|}}=0$
Maple

restart; 
ode66:=diff(y(x),x)-sqrt((abs((1-y(x))*y(x)*(1-a*y(x))))/(abs((1-x)*x*(1-a*x))))=0: 
dsolve(%,y(x));

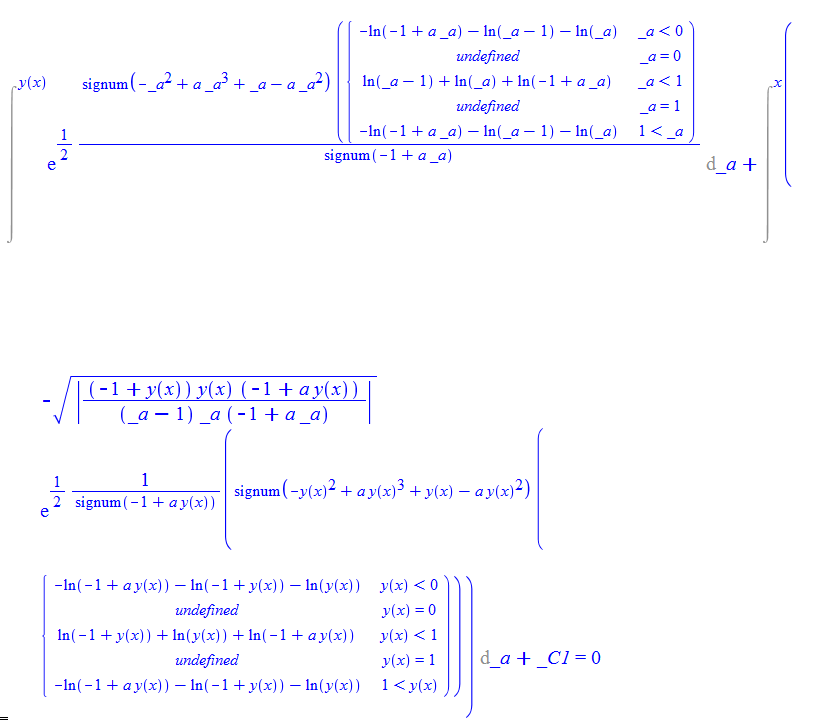
Mathematica

Clear["Global`*"]; 
ode66=D[y[x],x]-Abs[y[x]*(1-y[x])*(1-a*y[x])]^(1/2)/Abs[x*(1-x)*(1-a*x)]^(1/2)==0 
DSolve[%,y[x],x]//TraditionalForm

No answer after 10 minutes wait. Abort

#67

$y^{\prime }\left(x\right)-\sqrt{\frac{1+y{\left(x\right)}^4}{1+x^4}}=0$
Maple

restart; 
ode67:=diff(y(x),x)-sqrt((1-y(x)^4)/(1-x^4))=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode67 = D[y[x], x] - (1 - y[x]^4)^(1/2)/(1 - x^4)^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#68

$y^{\prime }\left(x\right)-\sqrt{\frac{1+by{\left(x\right)}^2+ay{\left(x\right)}^4}{1+b{x}^2+a{x}^4}}=0$
Maple

restart; 
ode68:=diff(y(x),x)-sqrt((1+b*y(x)^2+a*y(x)^4)/(1+b*x^2+a*x^4))=0: 
dsolve(%,y(x));

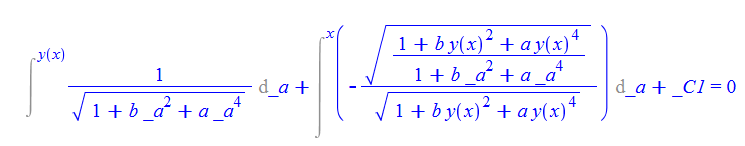
Mathematica

Clear["Global`*"]; 
ode68 = D[y[x],x]-((a*y[x]^4 + b*y[x]^2 + 1)/(a*x^4 + b*x^2 + 1))^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#69

$y^{\prime }\left(x\right)-\sqrt{(a_0+a_{1}x+a_2{x}^2+a_3{x}^3+a_4{x}^4)(b_0+b_{1}y\left(x\right)+b_{2}y{\left(x\right)}^2+b_{3}y{\left(x\right)}^3+b_{4}y{\left(x\right)}^4)}=0$
Maple

restart; 
ode69:=diff(y(x),x)-sqrt((a0+a1*x+a2*x^2+a3*x^3+a4*x^4)*(b0+b1*y(x)+b2*y(x)^2+b3*y(x)^3+b4*y(x)^4))=0: 
dsolve(%,y(x));

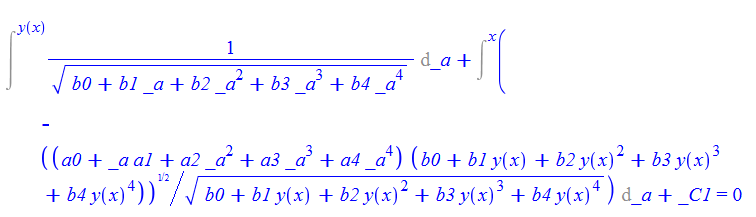
Mathematica

Clear["Global`*"]; 
ode69=D[y[x],x]-((b4*y[x]^4+b3*y[x]^3+b2*y[x]^2+b1*y[x]+b0)* 
       (a4*x^4+a3*x^3+a2*x^2+a1*x+a0))^(1/2)==0 
DSolve[%,y[x],x]//TraditionalForm

#70

$y^{\prime }\left(x\right)-\sqrt{\frac{a_0+a_{1}x+a_2{x}^2+a_3{x}^3+a_4{x}^4}{b_0+b_{1}y\left(x\right)+b_{2}y{\left(x\right)}^2+b_{3}y{\left(x\right)}^3+b_{4}y{\left(x\right)}^4}}=0$
Maple

restart; 
ode70:=diff(y(x),x)-sqrt((a0+a1*x+a2*x^2+a3*x^3+a4*x^4)/(b0+b1*y(x)+b2*y(x)^2+b3*y(x)^3+b4*y(x)^4))=0: 
dsolve(%,y(x));

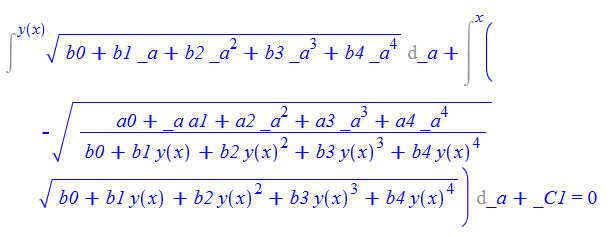
Mathematica

Clear["Global`*"]; 
ode70=D[y[x],x]-((a4*x^4 + a3*x^3 + a2*x^2 + a1*x + a0)/(b4*y[x]^4 + 
      b3*y[x]^3 + b2*y[x]^2 + b1*y[x] + b0))^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#71

$y^{\prime }\left(x\right)-\sqrt{\frac{b_0+b_{1}y\left(x\right)+b_{2}y{\left(x\right)}^2+b_{3}y{\left(x\right)}^3+b_{4}y{\left(x\right)}^4}{a_0+a_{1}x+a_2{x}^2+a_3{x}^3+a_4{x}^4}}=0$
Maple

restart; 
ode71:=diff(y(x),x)-sqrt((b0+b1*y(x)+b2*y(x)^2+b3*y(x)^3+b4*y(x)^4)/(a0+a1*x+a2*x^2+a3*x^3+a4*x^4))=0; 
dsolve(%,y(x));

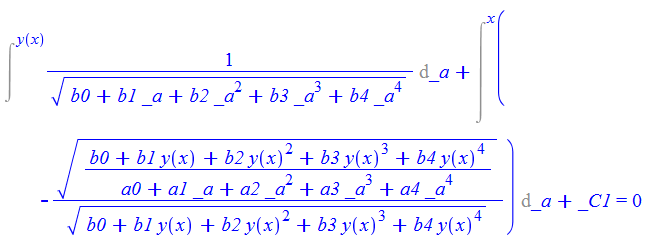
Mathematica

Clear["Global`*"]; 
ode71 = D[y[x],x] - ((b4*y[x]^4 + b3*y[x]^3 + b2*y[x]^2 + b1*y[x] + b0)/(a4*x^4 + 
         a3*x^3 + a2*x^2 + a1*x + a0))^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#72

$y^{\prime }\left(x\right)-R_1(x,\sqrt{a_0+a_{1}x+a_2{x}^2+a_3{x}^3+a_4{x}^4})R_2(y\left(x\right),\sqrt{b_0+b_{1}y\left(x\right)+b_{2}y{\left(x\right)}^2+b_{3}y{\left(x\right)}^3+b_{4}y{\left(x\right)}^4})=0$
Maple

restart; 
ode72:=diff(y(x),x)-R1(x,sqrt(a0+a1*x+a2*x^2+a3*x^3+a4*x^4))* 
R2(y(x),sqrt(b0+b1*y(x)+b2*y(x)^2+b3*y(x)^3+b4*y(x)^4))=0: 
dsolve(%,y(x));

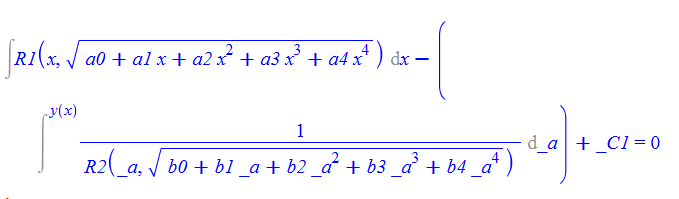
Mathematica

Clear["Global`*"]; 
ode72 = D[y[x], x] -R1[x, (a4*x^4 + a3*x^3 + a2*x^2 + a1*x + a0)^(1/2)]* 
    R2[y[x], (b4*y[x]^4 + b3*y[x]^3 + b2*y[x]^2 + b1*y[x] + b0)^(1/2)] == 0 
DSolve[%, y[x], x] // TraditionalForm

#73

$y^{\prime }\left(x\right)-{\left(\frac{a_0+a_{1}x+a_2{x}^2+a_3{x}^3+a_4{x}^4}{a_0+a_{1}y\left(x\right)+a_{2}y{\left(x\right)}^2+a_{3}y{\left(x\right)}^3}\right)}^{\frac{2}{3}}=0$
Maple

restart; 
ode73:=diff(y(x),x)-((a0+a1*x+a2*x^2+a3*x^3)/(a0+a1*y(x)+a2*y(x)^2+a3*y(x)^3))^(2/3)=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode73=D[y[x],x]-((a3*x^3+a2*x^2+a1*x+a0)/(a3*y[x]^3+a2*y[x]^2+a1*y[x]+a0))^(2/3)==0 
DSolve[%,y[x],x]//TraditionalForm

#74

$y^{\prime }\left(x\right)-f\left(x\right)\sqrt{(y\left(x\right)-a)(y\left(x\right)-b)}(y\left(x\right)-g\left(x\right))=0$
Maple

restart; 
ode74:=diff(y(x),x)-f(x)*sqrt((-a*y(x))*(-b*y(x)))*(y(x)-g(x))=0; 
dsolve(%,y(x));

Not solved

Mathematica

Clear["Global`*"]; 
ode74 = D[y[x], x]-f[x]*(y[x] - g[x])*((y[x] - a)*(y[x] - b))^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

Not solved

#75

$y^{\prime }\left(x\right)+e^x-e^{x-y\left(x\right)}=0$
Maple

restart; 
ode75:=diff(y(x),x)+exp(x)-exp(x-y(x))=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode75=D[y[x],x]-Exp[x-y[x]]+Exp[x]==0 
DSolve[%,y[x],x]//TraditionalForm

#76

$y^{\prime }\left(x\right)-b+a\ast \cos (y(x))=0$
Maple

restart; 
ode76:=diff(y(x),x)+b-a*cos(y(x))=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode76 = D[y[x], x] - a*Cos[y[x]] + b == 0 
DSolve[%, y[x], x] // TraditionalForm

#77

$y^{\prime }\left(x\right)-\cos (bx+ay\left(x\right))=0$
Maple

restart; 
ode77:=diff(y(x),x)-cos(b*x+a*y(x))=0: 
dsolve(%,y(x));

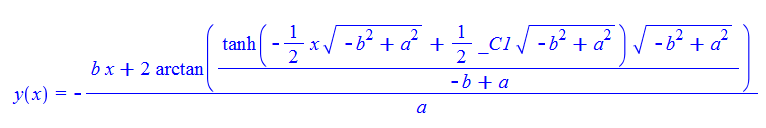
Mathematica

Clear["Global`*"]; 
ode77 = D[y[x], x] - Cos[a*y[x] + b*x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#78

$y^{\prime }\left(x\right)+a\sin (\alpha y\left(x\right)+\beta x)+b=0$
Maple

restart; 
ode78:=diff(y(x),x)+a* sin(alpha *y(x)+ beta*x)+b=0: 
dsolve(%,y(x));

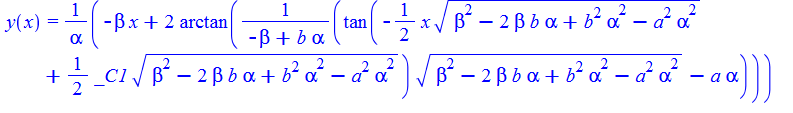
Mathematica

Clear["Global`*"]; 
ode78=D[y[x],x]+a*Sin[\[Alpha] y[x]+\[Beta]*x]+b==0 
DSolve[%,y[x],x]//TraditionalForm

#79

$y^{\prime }\left(x\right)+f\left(x\right)\cos \left(ay\left(x\right)\right)+g\left(x\right)\sin \left(ay\left(x\right)\right)+h\left(x\right)=0$
Maple

restart; 
ode79:=diff(y(x),x)+f(x)*cos(a*y(x))+g(x)*sin(a*y(x))+h(x)=0; 
dsolve(%,y(x));

No solution

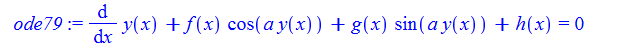
Mathematica

Clear["Global`*"]; 
ode79=D[y[x],x]+f[x]*Cos[a*y[x]]+g[x]*Sin[a*y[x]]+h[x]==0 
DSolve[%,y[x],x]//TraditionalForm

No solution

#80

$y^{\prime }\left(x\right)+f\left(x\right)\sin \left(y\left(x\right)\right)+(1-f^{\prime }\left(x\right))\cos \left(y\left(x\right)\right)-f^{\prime }\left(x\right)-1=0$
Maple

restart; 
ode80:=diff(y(x),x)+f(x)*sin(y(x))+(1-diff(f(x),x))*cos(y(x))-diff(f(x),x)-1=0; 
dsolve(%,y(x));

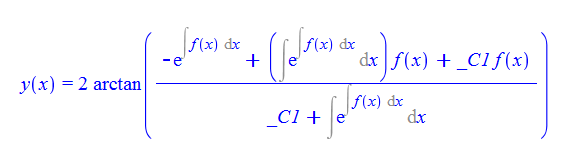
Mathematica

Clear["Global`*"]; 
ode80 = D[y[x], x] + f[x]*Sin[y[x]] + (1 - D[f[x], x])*Cos[y[x]] - 
   D[f[x], x] - 1 == 0 
DSolve[%, y[x], x] // TraditionalForm

No solution

#81

$y^{\prime }\left(x\right)+2\tan \left(y\left(x\right)\right)\tan \left(x\right)-1=0$
Maple

restart; 
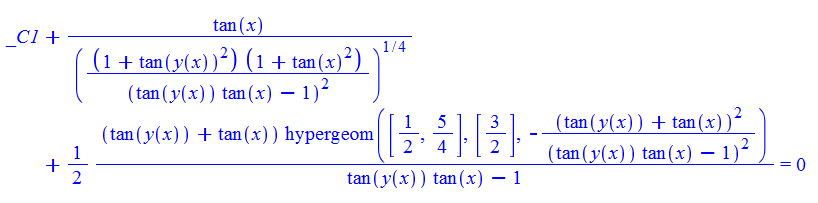
ode81:=diff(y(x),x)+2*tan(y(x))*tan(x)-1=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode81 = D[y[x], x] + 2*Tan[y[x]]*Tan[x] - 1 == 0 
DSolve[%, y[x], x] // TraditionalForm

No solution

#82

$y^{\prime }\left(x\right)-a(1+\tan {\left(y\left(x\right)\right)}^2)+\tan \left(y\left(x\right)\right)\tan \left(x\right)=0$
Maple

restart; 
ode82:=diff(y(x),x)-a*(1+tan(y(x))^2)+tan(y(x))*tan(x)=0; 
dsolve(%,y(x));

No solution

Mathematica

Clear["Global`*"]; 
ode82 = D[y[x], x] - a*(1 + Tan[y[x]]^2) + Tan[y[x]]*Tan[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

No solution

#83

$y^{\prime }\left(x\right)-\tan \left(xy\left(x\right)\right)=0$
Maple

restart; 
ode83:=diff(y(x),x)-tan(x*y(x))=0; 
dsolve(%,y(x));

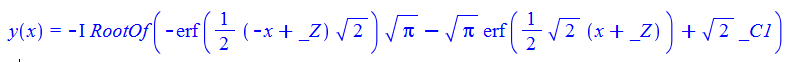
Mathematica

Clear["Global`*"]; 
ode83 = D[y[x], x] - Tan[x*y[x]] == 0 
DSolve[%, y[x], x] // TraditionalForm

No solution

#84

$y^{\prime }\left(x\right)-f(ax+by\left(x\right))=0$
Maple

restart; 
ode84:=diff(y(x),x)-f(a*x+b*y(x))=0: 
dsolve(%,y(x));

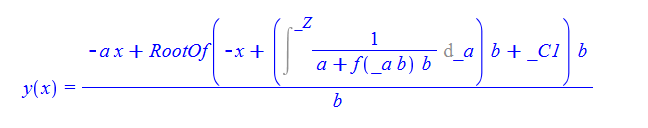
Mathematica

Clear["Global`*"]; 
ode84 = D[y[x], x] - f[a*x + b*y[x]] == 0 
DSolve[%, y[x], x] // TraditionalForm

#85

$y^{\prime }\left(x\right)-x^{a-1}f(\frac{x^a}{a}+\frac{y{\left(x\right)}^b}{b})y{\left(x\right)}^{1-b}=0$
Maple

restart; 
ode85:=diff(y(x),x)-x^(a-1)*y(x)^(1-b)*f(x^a/a+y(x)^b/b)=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode85 = D[y[x], x] - x^(a - 1)*y[x]^(1 - b)*f[x^a/a + y[x]^b/b] == 0 
DSolve[%, y[x], x] // TraditionalForm

#86

$y^{\prime }\left(x\right)-\frac{y\left(x\right)-xf(x^2+ay{\left(x\right)}^2)}{x+af(x^2+ay{\left(x\right)}^2)y\left(x\right)}=0$
Maple

restart; 
ode86:=diff(y(x),x)-((-x*f(x^2+a*y(x)^2)+y(x))/(x+a*f(x^2+a*y(x)^2)*y(x)))=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode86 = D[y[x],x]-(y[x]-x*f[x^2 + a*y[x]^2])/(x+a*y[x]*f[x^2+a*y[x]^2])==0 
DSolve[%, y[x], x] // TraditionalForm

#87

$y^{\prime }\left(x\right)-\frac{af\left(x^{c}y\left(x\right)\right)y\left(x\right)+c{x}^{a}y{\left(x\right)}^b}{bxf\left(x^{c}y\left(x\right)\right)-x^{a}y{\left(x\right)}^b}=0$
Maple

restart; 
ode87:=diff(y(x),x)-((a*f(x^c*y(x))*y(x)+c*x^a*y(x)^b)/(b*x*f(x^c*y(x))-x^a*y(x)^b))=0; 
dsolve(%,y(x));

no solution

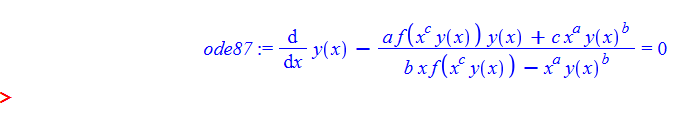
Mathematica

Clear["Global`*"]; 
ode87 = D[y[x],x]-(y[x]*a*f[x^c*y[x]]+c*x^a*y[x]^b)/(x*b*f[x^c*y[x]]-x^a*y[x]^b)== 0 
DSolve[%, y[x], x] // TraditionalForm

no solution

#88

$2y^{\prime }\left(x\right)-b-c{e}^{-2ax}-4ay\left(x\right)-3y{\left(x\right)}^2=0$
Maple

restart; 
ode88:=2*diff(y(x),x)-b-c*exp(-2*a*x)-4*a*y(x)-3*y(x)^2=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode88 = 2*D[y[x], x] - 3*y[x]^2 - 4*a*y[x] - b - c*Exp[-2*a*x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#89

$x{y}^{\prime }\left(x\right)-\sqrt{a^2-x^2}=0$
Maple

restart; 
ode89:=x*diff(y(x),x)-sqrt(a^2-x^2)=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode89 = x*D[y[x], x] - (a^2 - x^2)^(1/2) == 0 
DSolve[%, y[x], x] // TraditionalForm

#90

$x{y}^{\prime }\left(x\right)-x\sin \left(x\right)+y\left(x\right)=0$
Maple

restart; 
ode90:=x*diff(y(x),x)+y(x)-x*sin(x)=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode90 = x*D[y[x], x] + y[x] - x*Sin[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#91

$x{y}^{\prime }\left(x\right)-y\left(x\right)-\frac{x}{\ln \left(x\right)}=0$
Maple

restart; 
ode91:=x*diff(y(x),x)-y(x)-x/log(x)=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode91 = x*D[y[x], x] - y[x] - x/Log[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#92

$x{y}^{\prime }\left(x\right)-y\left(x\right)-x^2\sin \left(x\right)=0$
Maple

restart; 
ode92:=x*diff(y(x),x)-y(x)-x^2*sin(x)=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode92 = x*D[y[x], x] - y[x] - x^2*Sin[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#93

$x{y}^{\prime }\left(x\right)-y\left(x\right)-\frac{x\cos (\ln (\ln \left(x\right)))}{\ln \left(x\right)}=0$
Maple

restart; 
ode93:=x*diff(y(x),x)-y(x)-(x*cos(log(log(x))))/(log(x))=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode93 = x*D[y[x], x] - y[x] - x*Cos[Log[Log[x]]]/Log[x] == 0 
DSolve[%, y[x], x] // TraditionalForm

#94

$x{y}^{\prime }\left(x\right)+b{x}^n+ay\left(x\right)=0$
Maple

restart; 
ode94:=x*diff(y(x),x)+b*x^n+a*y(x)=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode94 = x*D[y[x], x] + a*y[x] + b*x^n == 0 
DSolve[%, y[x], x] // TraditionalForm

#95

$x{y}^{\prime }\left(x\right)+x^2+y{\left(x\right)}^2=0$
Maple

restart; 
ode95:=x*diff(y(x),x)+x^2+y(x)^2=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode95 = x*D[y[x], x] + y[x]^2 + x^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#96

$x{y}^{\prime }\left(x\right)-y{\left(x\right)}^2+1=0$
Maple

restart; 
ode96:=x*diff(y(x),x)+1-y(x)^2=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode96 = x*D[y[x], x] - y[x]^2 + 1 == 0 
DSolve[%, y[x], x] // TraditionalForm

#97

$x{y}^{\prime }\left(x\right)+b{x}^2-y\left(x\right)+ay{\left(x\right)}^2=0$
Maple

restart; 
ode97:=x*diff(y(x),x)+b*x^2-y(x)+a*y(x)^2=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode97 = x*D[y[x], x] + a*y[x]^2 - y[x] + b*x^2 == 0 
DSolve[%, y[x], x] // TraditionalForm

#98

$x{y}^{\prime }\left(x\right)+c{x}^{2b}-by\left(x\right)+ay{\left(x\right)}^2=0$
Maple

restart; 
ode98:=x*diff(y(x),x)+c*x^(2*b)-b*y(x)+a*y(x)^2=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode98 = x*D[y[x], x] + a*y[x]^2 - b*y[x] + c*x^(2*b) == 0 
DSolve[%, y[x], x] // TraditionalForm

#99

$x{y}^{\prime }\left(x\right)-c{x}^{\beta }-by\left(x\right)+ay{\left(x\right)}^2=0$
Maple

restart; 
ode99:=x*diff(y(x),x)-c*x^beta-b*y(x)+a*y(x)^2=0; 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode99 = x*D[y[x], x] + a*y[x]^2 - b*y[x] - c*x^\[Beta] == 0 
DSolve[%, y[x], x] // TraditionalForm

#100

$x{y}^{\prime }\left(x\right)+a+xy{\left(x\right)}^2=0$
Maple

restart; 
ode100:=x*diff(y(x),x)+a+x*y(x)^2=0: 
dsolve(%,y(x));

Mathematica

Clear["Global`*"]; 
ode100 = x*D[y[x], x] + x*y[x]^2 + a == 0 
DSolve[%, y[x], x] // TraditionalForm

#101

$x{y}^{\prime }\left(x\right)-y\left(x\right)+xy{\left(x\right)}^2=0$
Maple

restart; 
ode101:=x*diff(y(x),x)-y(x)+x*y(x)^2=0: 
dsolve(%,y(x));